HPC User Report from F. Wein (Professorship for Mathematical Optimization)

Within the Cluster of Excellence Engineering of Advanced Materials (EAM) we optimized phononic band gaps in a collaborative project with the Chair of Materials Science and Engineering for Metals (C. Körner), FAU.

Standard topology optimization fails to produce self carrying single phase structures. To this end we developed a shape optimization approach based on topology optimization code.

The obtained designs show relative band gaps up to a value of 6 (upper minus lower frequencies of the gap divided by lower frequency).

Motivation and problem definition

Phononic band gap structures suppress mechanical waves. They belong to meta-materials, which are designed materials with properties not found in nature.

Methods and codes

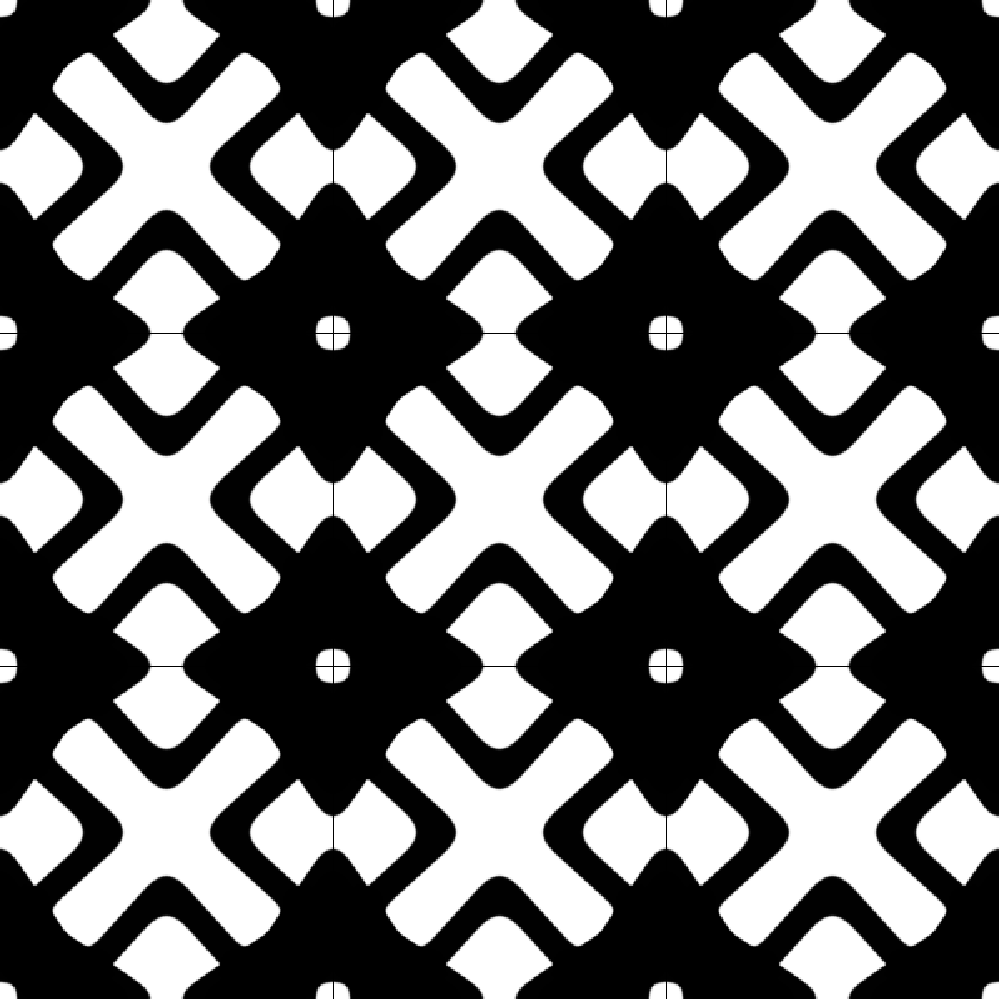The structural optimization approach is gradient based optimization based on a complex valued (Floquet-Bloch analysis) eigenvalue problems solved by the finite element method. The optimization solver is commercial (SNOPT), the structural optimization and FEM-Code is self developed (CFS++), the eigenvalue solver is open source (ARPACK).

Band gap problems are known to be non-smooth due to multiple and switching modes. Albeit we tried to compensate these issues, the highly complex non-linear problem formulation with approx. 72 non-smooth eigenvalue based constraints makes it difficult to solve the problems.

However, these issues could be compensated by a multiple-shot approach based on the RRZE HPC computational power (Woody).

Results

New structures exhibiting phononic band gap behavior have been found.

Outreach

Wormser, M., Wein, F., Stingl, M., & Körner, C. (2017). Design and additive manufacturing of 3D phononic band gap structures based on gradient based optimization. Materials, 10(10), 1125. DOI:10.3390/ma10101125. Supported by the Cluster of Excellence Engineering of Advanced Materials (DFG).

Researcher’s Bio and Affiliation

Dr. Fabian Wein studied Electrical Engineering and Computation Engineering, his research interests are focused on structural engineering, e.g. optimization of meta-materials or optimization of multiphysic problems.